Getting to Know: Priyam Patel       

Priyam Patel

Imagine a surface that looks like a hollow doughnut. The “skin” of the doughnut has no thickness and is made of stretchy, flexible material. “Some of my favorite mathematical problems deal with objects like this—surfaces and curves or loops on such surfaces,” said Priyam Patel, assistant professor of mathematics, who joined the Math Department in 2019. “I like how artistic and creative my work feels, and it’s also very tangible since I can draw pictures representing different parts of a problem I’m working on.”  

Patel works in geometry and topology. The two areas differ in that geometry focuses on rigid objects where there is a notion of distance, while topological objects are much more fluid. Patel likes studying a geometrical or topological object extensively so that she’s able to get to know the space, how it behaves, and what sort of phenomena it exhibits. In her research, Patel’s goals are to study and understand curves on surfaces, symmetries of surfaces, and objects called hyperbolic manifolds and their finite covering spaces. Topology and geometry are used in a variety of fields, including data analysis, neuroscience, and facial recognition technology. Patel’s research doesn’t focus on these applications directly since she works in pure mathematics.  

Challenges as a Minority 

Patel became fascinated with mathematics in high school while learning to do proofs. She was fortunate to have excellent high school math teachers, who encouraged her to consider majoring in math in college. “When I was an undergraduate at New York University (NYU), I had a female professor for multivariable calculus who spent a lot of time with me in office hours and gave me challenging problems to work on,” said Patel. “She was very encouraging and had a huge impact on me.”

As a woman of color, Patel often felt out of place in many of her classes at NYU. Later, she was one of a handful of women accepted into a Ph.D. program at Rutgers University. Unfortunately, these experiences led to strong feelings of “impostor syndrome” for her as a graduate student. Eventually, she overcame them and learned to celebrate her successes, focusing on the joy that mathematics brings to her life. She has also worked to find a community of mathematicians to help support her through the tough times. “I’ve received a lot of encouragement from friends and mentors both in and outside of my math community,” she said. “I feel especially fortunate to have connected with strong women mentors in recent years.”   

Mentors and Outside Interests  

Feng Luo, professor of mathematics at Rutgers, was Patel’s Ph.D. advisor, and he played an active role in the early years of her math career. “Talking about math with Dr. Luo is always a positive experience, and his encouragement has been pivotal to my success as a mathematician,” said Patel. Another mentor is Alan Reid, chair and professor of the Department of Mathematics at Rice University. Patel notes there are many aspects to being a mathematician outside of math itself, and these mentors have helped her navigate her career and offered support, encouragement, and advice.  

Patel loves mathematics but makes time for other things in life. She enjoys rock climbing, yoga, dancing, and painting. Music is also a huge part of her life, and she sings and plays the guitar.

Future Research

Patel is currently working on problems concerning groups of symmetries of certain surfaces. Specifically, she has been studying the mapping class groups of infinite-type surfaces, which is a new and quickly growing field of topology. “It’s quite exciting to be at the forefront of it. I would like to tackle some of the biggest open problems in this area in the next few years, such as producing a Nielsen-Thurston type classification for infinite-type surfaces,” she said. She is also interested in the work of Ian Agol, professor of mathematics at Berkeley, who won a Breakthrough Prize in 2012 for solving an open problem in low-dimensional topology. Patel would like to build on Agol’s work in proving a quantitative version of his results. Other areas she’d like to explore are the combinatorics of 3-manifolds and the theory of translation surfaces.

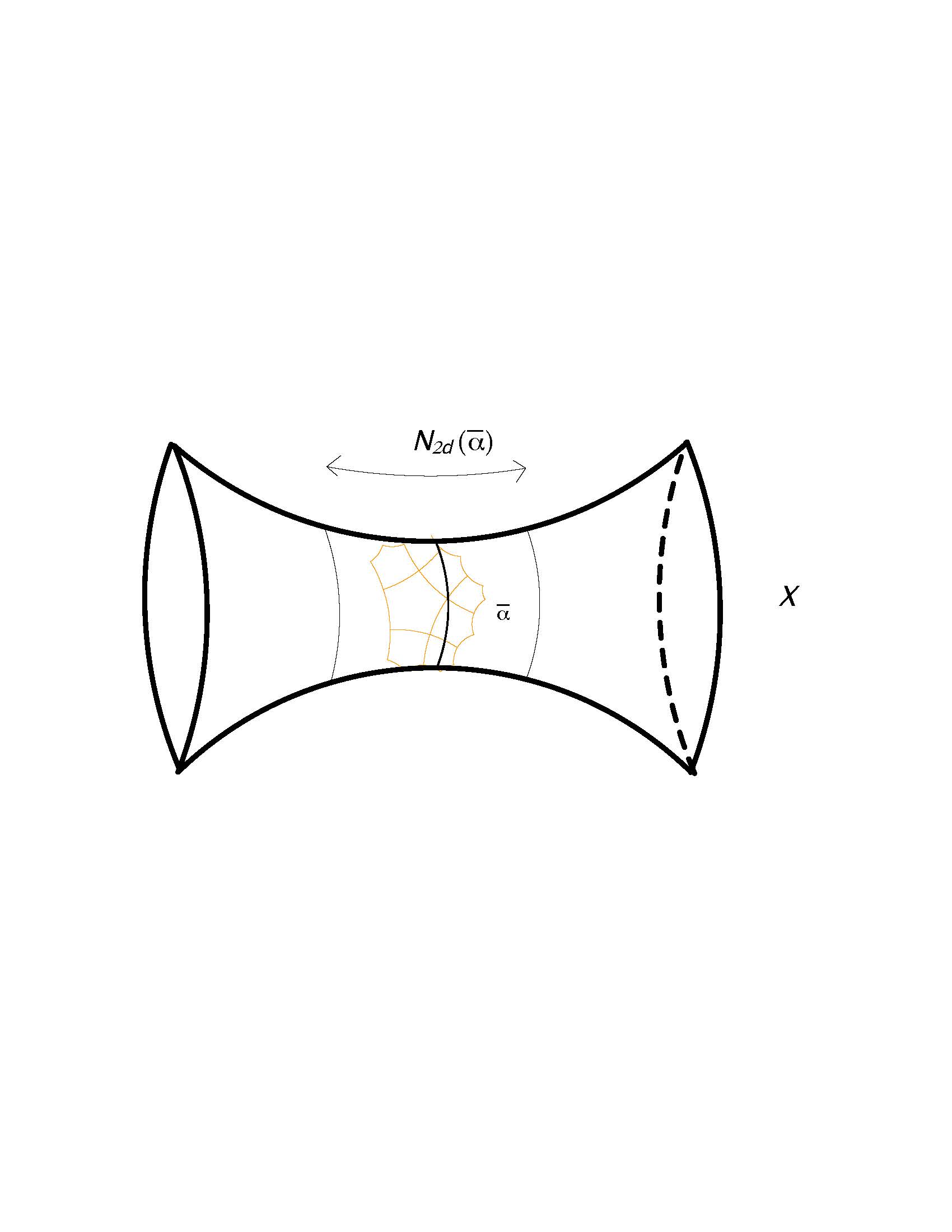
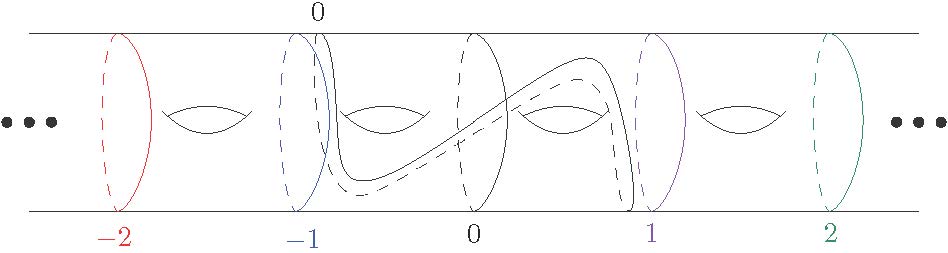
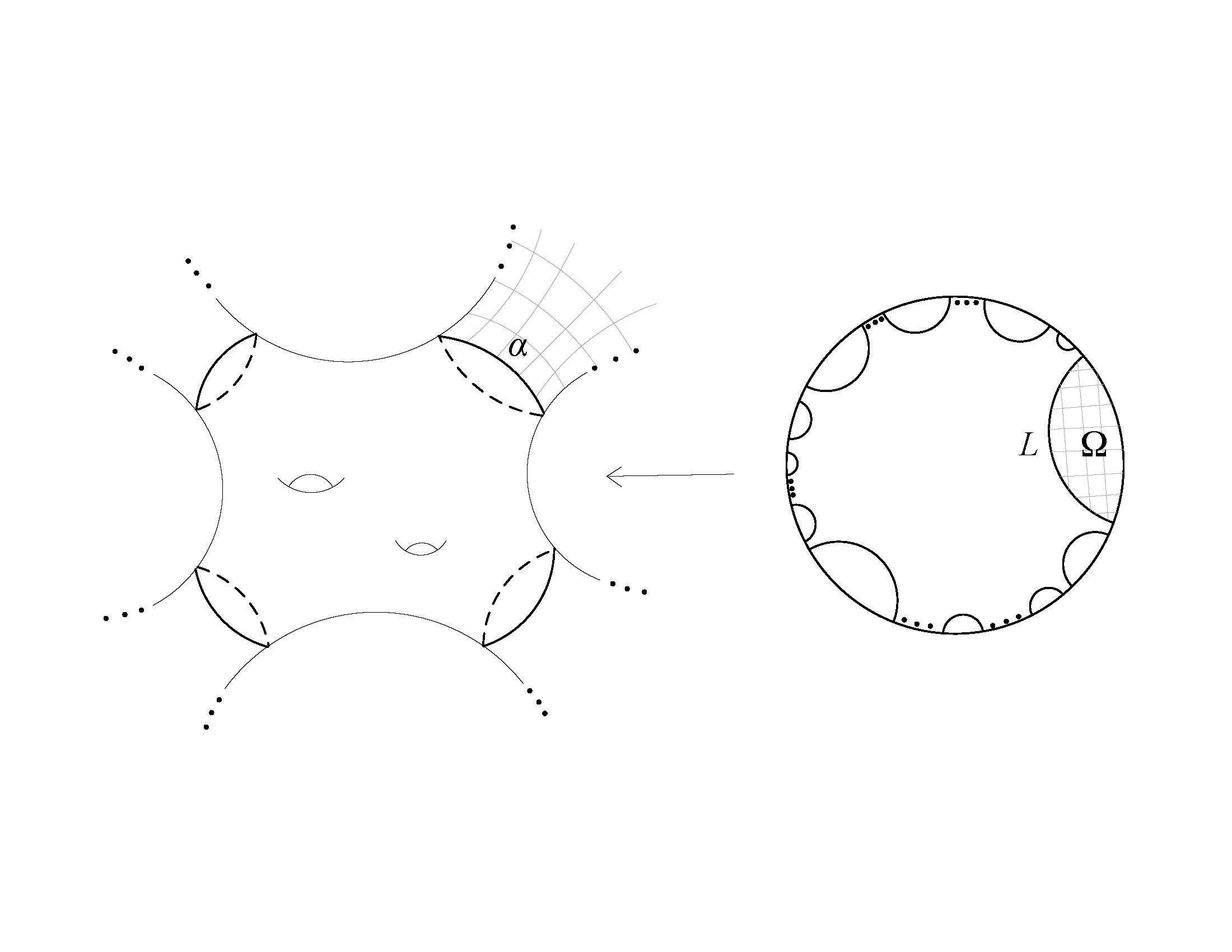
Below are some mathematical images created by Patel in her research: 

 Patel was featured in two separate articles in Quanta Magazine. Here are the links to the two articles:

February: https://www.quantamagazine.org/mathematics-as-a-team-sport-20200331/  
April: https://www.quantamagazine.org/how-has-coronavirus-affected-mathematics-20200428/