Equilibrium

Effective properties of a laminate are defined by the type of equilibrium in the medium. Here we derive these properties. Assume that the materials are linear, that is that the constitutive relation in $i-$th material is

$$u_i= D_i v_i$$

Here the vectors $v_i$ and $u_i$ represent the ``prime'' and ``dual'' fields, respectively, joined by the tensor of material's properties $D_i$. These fields are constant if the composite is uniformly loaded or if the width of layers does to zero.

Examples

1. For conducting composite, the constitutive relations is the Ohm's law:
   
   $$j= \sigma e$$
   
   
   where $j$ is the current (dual variable), $e$ is the electrical field (prime variable), and $\sigma$ is the conductivity tensor. Of course, we may reverse the Ohm's law:
   
   $$e= {1 \over \sigma} j;$$
   
   
   then $e$ is a dual and $j$ is a prime variable.

2. For elastic materials, the constitutive relation is the Hook's law
   
   $$\tau= C:\epsilon$$
   
   
   where $\tau$ is the symmetric second-rank stress tensor, $\epsilon$ is the symmetric second-rank strain tensor, $C$ is the fourth-rank tensor of elastic stiffness, (:) is the scalar product in the tensor space.

Let us derive the effective properties of a two-component laminate. It is characterized by a normal ${ n}$ and by volume fractions $m_{1}$ and $m_{2}$ of subdomains $\Omega_1$ and $\Omega_2$ that are occupied by the materials with tensor properties $D_1$ and $D_2$. The composite is submerged into a uniform field ${ v}_{0}$.

The fields ${u, v}$ in the laminates are piecewise constant:

$$v(x)= \left\{\begin{array}{rll} v_1 & \mbox{ if } & x\in \... ...a_1 \\ v_2 & \mbox{ if } & x\in \Omega_2 \end{array}\right.$$

where $v_i$ = constant($x$).

The effective tensor of laminate $D_{\mbox{\footnotesize {lam}}}$ joins the averaged fields

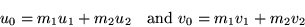$$u_0= m_1 { u}_1 + m_2 { u}_2 \quad \mbox{and } v_0= m_1 { v}_1 + m_2 { v}_2$$ (1)

as follows:

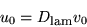$${ u}_0= D_{\mbox{\footnotesize {lam}}} { v}_0$$ (2)

where $D_{\mbox{\footnotesize {lam}}}$ is the effective tensor of laminates which we are determining here.